how to find wavelength with only frequency

All electromagnetic radiation is calorie-free, and information technology occurs over an extremely wide range of wavelengths, from high-energy gamma waves with shorter wavelengths to low-free energy radio waves with longer wavelengths. But the human centre can detect just a small-scale portion of the radiation, and that portion is referred to as visible calorie-free. In an electromagnetic spectrum, the visible spectrum lies in between the infrared spectrum and the UV spectrum. Visible light ranges betwixt a wavelength of 400 nm and 700 nm. The homo eye cannot find other electromagnetic radiations every bit the radiations has either large or minor wavelengths and is out of biological limitations.

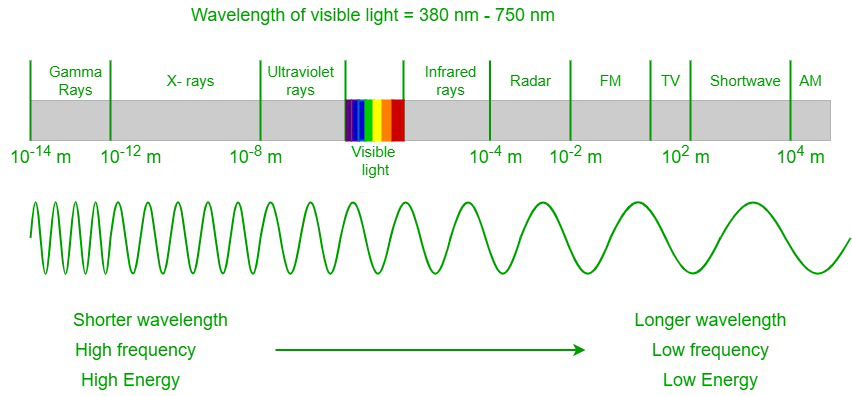
Electromagnetic spectrum

When a visible spectrum travels through a prism, the light gets separated into a spectrum of colors of different wavelengths. The violet color has the shortest wavelength of around 380 nm, and the red color has the longest wavelength of around 700 nm. Our eyes tin can detect the outer-most layer of the sun, the corona, in visible light.

Wavelength

A wavelength is i of the backdrop of a wave and is defined as the distance betwixt the 2 successive crests or troughs of a wave, where a crest is the highest bespeak of the wave, and a trough is the everyman point of the moving ridge. Since wavelength is a altitude or length between 2 points, it is measured in meters, centimeters, millimeters, micrometers, etc. It is denoted by the symbol Lambda 'λ'.

Wave

Frequency

Frequency (f) is divers as the total number of wave cycles or oscillations produced per unit of time. Frequency is measured in terms of Hertz (Hz) or due south^-1.

The formula for the frequency:

Frequency (f) = 1/period(T)

f = one/T

A period is defined equally the time taken to complete an oscillation.
From the equation of frequency, we can conclude that the frequency of a moving ridge is inversely proportional to its menstruum.
1 Hertz = 1 oscillation/second

Wave velocity

The velocity of a wave or moving ridge velocity is divers as the distance traveled by the wave in a unit of time. The S.I. unit of wave velocity is ms^-1.

Lite travels with a speed in the vacuum of 29,97,92,458 m/s, i.east., approximately 3 × ten^viii k/s, and it is represented by the symbol c.

Wavelength of the light

We know that light possesses the characteristics of both a moving ridge and a particle. So, the wavelength of a low-cal wave is given as;

λ = $\frac{c}{f}$

Where λ is the wavelength of light

c is the velocity of light and

f is the frequency of the light

The energy of a photon is given as,

Eastward = h × f = $\frac{hc}{\lambda}$

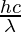
Where E is the free energy of a photon

h is the Planck'due south constant i.east., h = 6.64 × ten^-34 joule-second

Wavelength, Frequency, and Energy of the visible light spectrum

Color	Wavelength	Frequency	The free energy of a photon
Violet	380 – 450 nm	668-789 THz	2.75 – 3.26 eV
Blue	450-495 nm	606-668 THz	2.fifty – ii.75 eV
Green	495-570 nm	526-606 THz	ii.17 – 2.50 eV
Yellow	570-590 nm	508-526 THz	2.ten – 2.17 eV
Orange	590-620 nm	484-508 THz	2.00 – two.10 eV
Carmine	620-750 nm	400-484 THz	1.65 – 2.00 eV

Sample Problems

Problem 1: Summate the wavelength of the visible light with a frequency of 5.36 × 10¹⁴ Hz.

Solution:

Given the frequency of light = 5.36 × 10¹⁴ Hz

We know, that the velocity of light (c) = 3 × 10^eight yard/southward

Now, the wavelength of light (λ) = $\frac{c}{f}$

⇒ λ = $\frac{3\times10^{8}}{5.36\times10^{14}}$

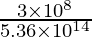
⇒ λ = v.60 × x^-7 m

Hence, the wavelength is 5.60 × 10^-7 g

Problem ii: If a microwave oven emits microwave free energy of 1.64 × 10^-24J, then calculate the wavelength of the microwave emitted.

Solution:

Given information,

The energy of microwave emitted = 1.64 × x^-24 J

Nosotros know, that the energy of a photon = $\frac{hc}{\lambda}$

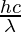
h = 6.64 × 10^-34 joule-second

⇒ 1.64 × x^-24 = $\frac{6.64×10^{-34}×3×10^{8}}{λ}$

⇒ λ = $\frac{6.64\times10^{-34}\times3\times10^{8}}{1.64\times10^{-24}}$

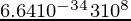
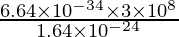
⇒ λ = 12.146 × ten^-ii yard = 12.15 cm

Hence, the wavelength of the microwave emitted is 12.xv cm.

Trouble 3: If a radio station broadcasts at a frequency of 555 kHz, then calculate the wavelength of radio waves emitted.

Solution:

Given,

Frequency of radio waves = 555 KHz

Nosotros know, that the velocity of calorie-free (c) = iii × ten^viii k/southward

Now, the wavelength of light (λ) = $\frac{c}{f}$

⇒ λ = $\frac{3\times10^{8}}{555\times10^{3}}$

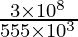
⇒ λ = 540 yard

Hence the wavelength of radio waves emitted is 540 1000.

Problem iv: Calculate the wavelength of yellow calorie-free emitted from a sodium lamp at a frequency of 5.15 × ten¹⁴ Hz.

Solution:

Given,

The frequency of xanthous light = 5.15 × 10^fourteen Hz

Nosotros know, that the velocity of light (c) = iii × 10⁸ m/due south

Now, the wavelength of light (λ) = $\frac{c}{f}$

⇒ λ = $\frac{3\times10^{8}}{5.15\times10^{14}}$

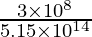
⇒ λ = 582.v × ten^-9 m = 582.5 nm

Hence, the wavelength of the yellow lite is 582.5 nm.

Problem 5: Calculate the wavelength of a photon with an free energy of 3.35 × 10^-19 Joules.

Solution:

Given,

The free energy of a photon = 3.35 × 10^-19 Joules.

We know, that the free energy of a photon = $\frac{hc}{\lambda}$

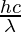
h = half dozen.64 × x^-34 joule-2d

⇒ iii.35 × 10^-19 = $\frac{6.64\times10^{-34}\times3\times10^{8}}{\lambda}$

⇒ λ = $\frac{6.64\times10^{-34}\times3\times10^{8}}{3.35\times10^{-19}}$

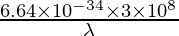
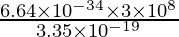
⇒ λ= 5.94 × 10^-7 m = 594 nm

Hence, the wavelength of the photon is 594 nm.

Trouble 6: The broadcasting frequency of a radio station is 101 MHz. What volition exist the wavelength of the wave if the broadcast wave is an electromagnetic wave?

Solution:

Given data, Frequency of the wave = 101 MHz = 101 × 10^{half dozen}Hz

Speed of low-cal = 3 × 10⁸m/s

Now, the wavelength of calorie-free (λ) = c/f

⇒ λ = (3 × ten⁸)/(101 × x⁶)

⇒ λ = 2.97 one thousand

Hence, the wavelength of the broadcast wave is 2.97m